
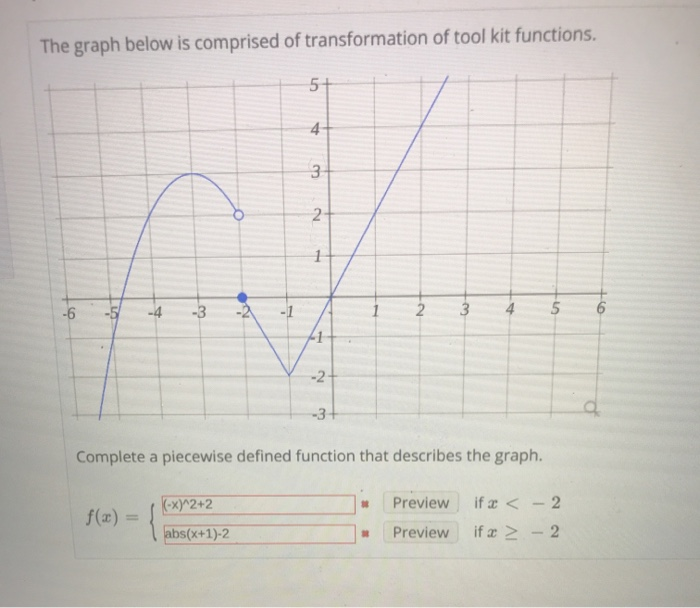
These are infinitely differentiable, but analyticity holds only piecewise. For the numerical integration is O (1e-11) and in the derivative it is O (1e-14).Plot of the piecewise linear function f ( x ) = and some other common Bump functions. The error in calculating the Fourier coefficients is O (1e-8). Limits of Piecewise-Defined Functions Quick Overview If x is approaching one of the transition points of the function, then you have to check both one-sided limits. In the Fourier coefficients calculations case, it depends on the function and size of the chosen integration interval. Area of a Triangle: Intuitive Discovery Getting to Know GeoGebra Area of a Circle Area of a Trapezoid: Intuitive Discovery Mean, Median, Mode, Range: Quick Check. Solution We graph the line y x+3, except we leave a hole at the point ()3, 6, since 3 is deleted from the subdomain of the top rule. Note that in numerical analysis, errors are obtained due to the particular methods and the limitation of arithmetic computer as well. (Section 1.5: Piecewise-Defined Functions Limits and Continuity in Calculus) 1.5.5 Example 3 (Graphing a Piecewise-Defined Function with a Removable Discontinuity) Graph f, if fx() x +3, x 3 7, x 3. § Solution We graph the line y x+3, except we leave a hole at the point ()3, 6, since 3 is deleted from the subdomain of the top rule. Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals. Pan the graph (move it) by holding the Shift key and dragging the graph with the mouse. Use the following steps in Graph mode: 1) Enter the equation for the first interval. Sketching a piece-wise function means sketching the graphs of several functions with applied domains. To calculate the primitive function: numerical integration methods seen in the numerical methods section are applied. What the calculator can do On this page you can get various actions with a piecewise-defined function, as well as for most services - get the detailed solution. (Section 1.5: Piecewise-Defined Functions Limits and Continuity in Calculus) 1.5.5 Example 3 (Graphing a Piecewise-Defined Function with a Removable Discontinuity) Graph f, if fx() x +3, x 3 7, x 3. A piecewise function is a function built from pieces of different.

The slash is merely the division symbol and is entered simply by pressing the key. When you are finished, they will look like this: Notice the slash and the inequality in parentheses after each function. Using the key, you enter the two pieces, one as Y1 and the other as Y2. To calculate the derivative of the function: uses severeal numerical methods to derivate. PIECEWISE-DEFINED FUNCTIONSON A GRAPHING CALCULATOR. In Fourier coefficients case, there are several methods to make the calculations programmed here by the owners of Mathstools. In the piecewise function case, operate as followsģ) Write the first function in the text box with the label functionĤ)Ğnter the upper sub-range in the text box labeled Subinterval 1.ĥ) Write the function as defined in the first sub-interval in the text box labeled subinterval 1įor example suppose we have the piecewise function
Calculations accuracy depends largely of size-interval introduzed and number of selected coefficients to calculate.ġ) Write the lower end of the range in the text box labeled Limit inf.Ģ)Ğnter the upper range in the text box labeled Limit Sup.ģ) Write the function in the text box with the label function


 0 kommentar(er)
0 kommentar(er)
